\begin{frame}
  \frametitle{Average Value of a Function}
  
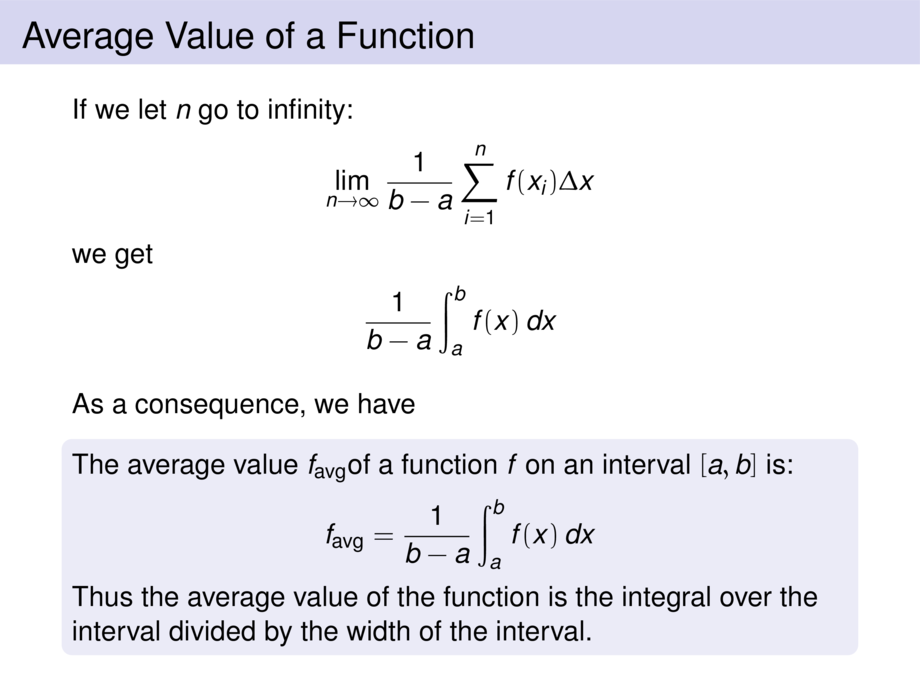
  If we let $n$ go to infinity:
  \begin{talign}
    \lim_{n \to \infty} \frac{1}{b-a}\sum_{i = 1}^n f(x_i)\Delta x
  \end{talign}  
  we get\pause
  \begin{talign}
    \frac{1}{b-a} \int_a^b f(x) \, dx
  \end{talign}
  \pause
  
  As a consequence, we have
  \begin{block}{}
    The average value $f_{\text{avg}}$of a function $f$ on an interval $[a,b]$ is:
    \begin{talign}
      f_{\text{avg}} = \frac{1}{b-a} \int_a^b f(x) \, dx
    \end{talign}
    Thus the average value of the function is the integral over the interval divided by the width of the interval.
  \end{block}
\end{frame}