\begin{frame}
  \frametitle{Review - Midterm Exam 3}

  \begin{exampleblock}{}
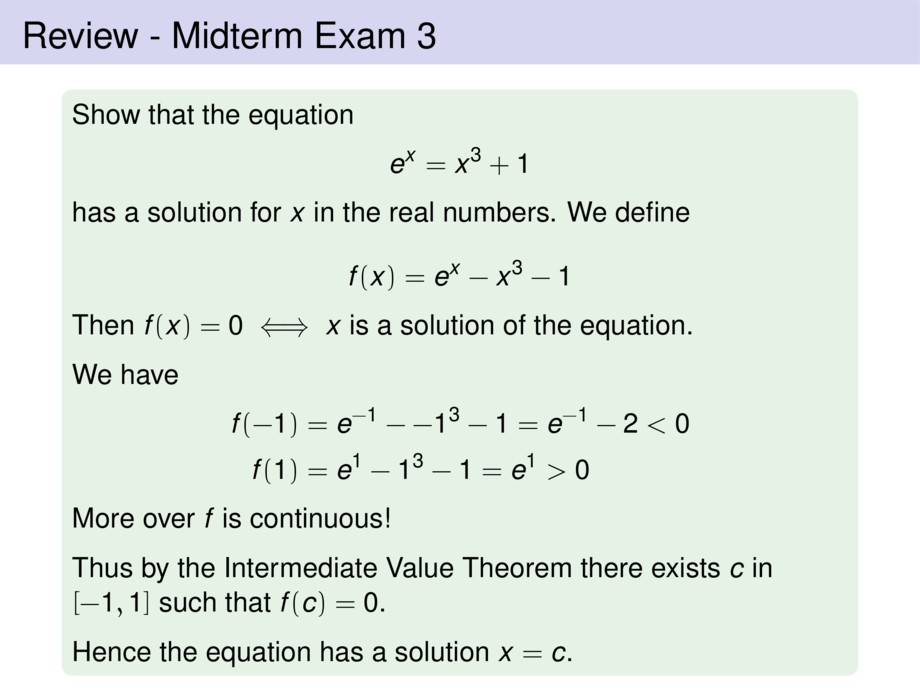
    Show that the equation
    \begin{talign}
      e^x = x^3 + 1
    \end{talign}
    has a solution for $x$ in the real numbers.
    \pause\medskip
    We define
    \begin{talign}
      f(x) = e^x - x^3 - 1
    \end{talign}
    \pause
    Then $f(x) = 0 \iff $ $x$ is a solution of the equation.
    \pause\medskip
    
    We have
    \begin{talign}
      f(-1) &= e^{-1} - {-1}^3 - 1 = e^{-1} - 2 < 0 \\
      \mpause[1]{f(1) &= e^{1} - {1}^3 - 1 = e^{1} > 0}
    \end{talign}
    \pause\pause
    More over $f$ is continuous!
    \pause\medskip
    
    Thus by the Intermediate Value Theorem there exists $c$ in $[-1,1]$ such that $f(c) = 0$.
    \pause\medskip
    
    Hence the equation has a solution $x = c$.
  \end{exampleblock}
\end{frame}