\begin{frame}
  \frametitle{Exponential Radioactive Decay}
  
  \begin{exampleblock}{}
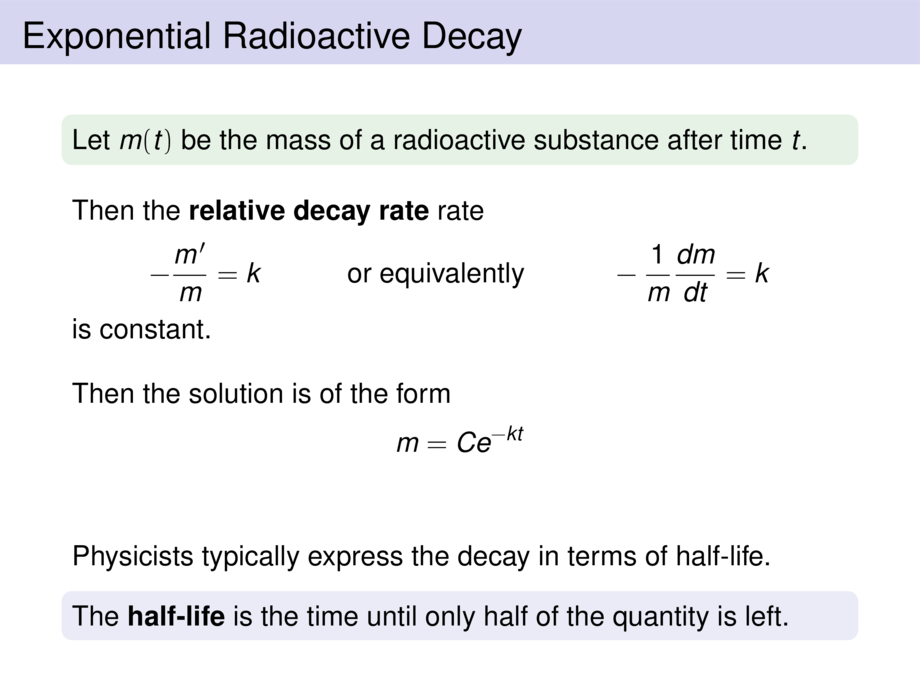
    Let $m(t)$ be the mass of a radioactive substance after time $t$.
  \end{exampleblock}
  \pause\medskip
  
  Then the \emph{relative decay rate} rate 
  \begin{talign}
    -\frac{m'}{m} = k &&\mpause[1]{\text{ or equivalently }}&&\mpause[1]{-\frac{1}{m}\frac{dm}{dt} = k}
  \end{talign}
  is constant.
  \pause\pause\bigskip

  Then the solution is of the form
  \begin{talign}
    m = Ce^{-kt}
  \end{talign}  
  \pause\bigskip

  Physicists typically express the decay in terms of half-life.
  \pause
  \begin{block}{}
    The \emph{half-life} is the time until only half of the quantity is left.
  \end{block}
\end{frame}