\begin{frame}
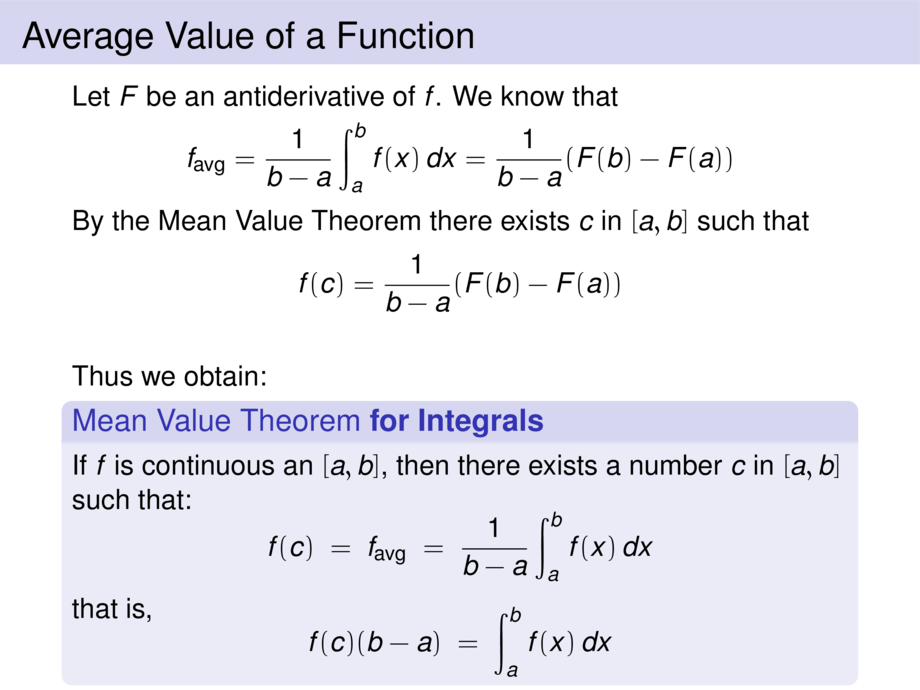
  \frametitle{Average Value of a Function}
  
  Let $F$ be an antiderivative of $f$. We know that
  \begin{talign}
    f_{\text{avg}} = \frac{1}{b-a} \int_a^b f(x) \, dx
    \mpause[1]{= \frac{1}{b-a} (F(b) - F(a))}
  \end{talign}
  \pause\pause
  By the Mean Value Theorem there exists $c$ in $[a,b]$ such that
  \begin{talign}
    f(c) = \frac{1}{b-a} (F(b) - F(a))
  \end{talign}
  \pause\medskip

  Thus we obtain:
  \begin{block}{Mean Value Theorem \emph{for Integrals}}
    If $f$ is continuous an $[a,b]$, then there exists a number $c$ in $[a,b]$ such that:\vspace{-1ex}
    \begin{talign}
      f(c) \;=\; f_{\text{avg}} \;=\; \frac{1}{b-a} \int_a^b f(x) \, dx
    \end{talign}
    \pause
    that is,\vspace{-2.2ex}
    \begin{talign}
      f(c) (b-a) \;=\; \int_a^b f(x) \, dx 
    \end{talign}
  \end{block}
  \vspace{10cm}
\end{frame}