\begin{frame}
  \frametitle{The Substitution Rule for Indefinite Integrals}

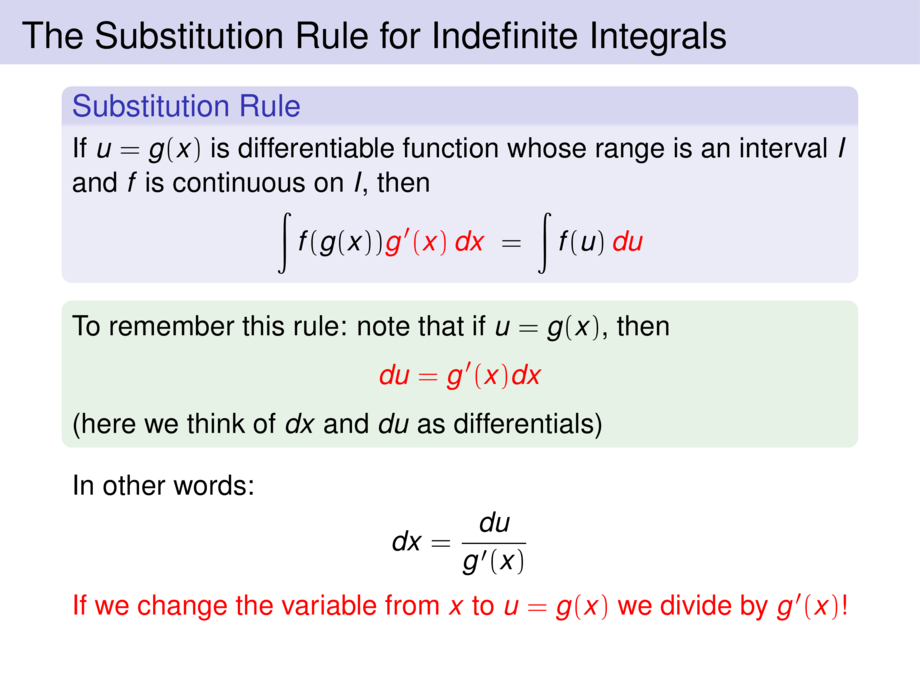
  \begin{block}{Substitution Rule}
    If $u = g(x)$ is differentiable function whose range is an interval $I$
    and $f$ is continuous on $I$, then
    \begin{talign}
      \int f(g(x)) \alert<2->{g'(x) \,dx} \;=\; \int f(u) \,\alert<2->{du}
    \end{talign}
  \end{block}
  \pause
  
  \begin{exampleblock}{}
  To remember this rule: note that if $u = g(x)$, then
  \begin{talign}
    \alert{du = g'(x) dx}
  \end{talign}
  (here we think of $dx$ and $du$ as differentials)
  \end{exampleblock}
  \pause\medskip
  
  In other words:
  \begin{talign}
    dx = \frac{du}{g'(x)}
  \end{talign}
  \alert{If we change the variable from $x$ to $u = g(x)$ we divide by $g'(x)$!}
  \vspace{10cm}
\end{frame}