



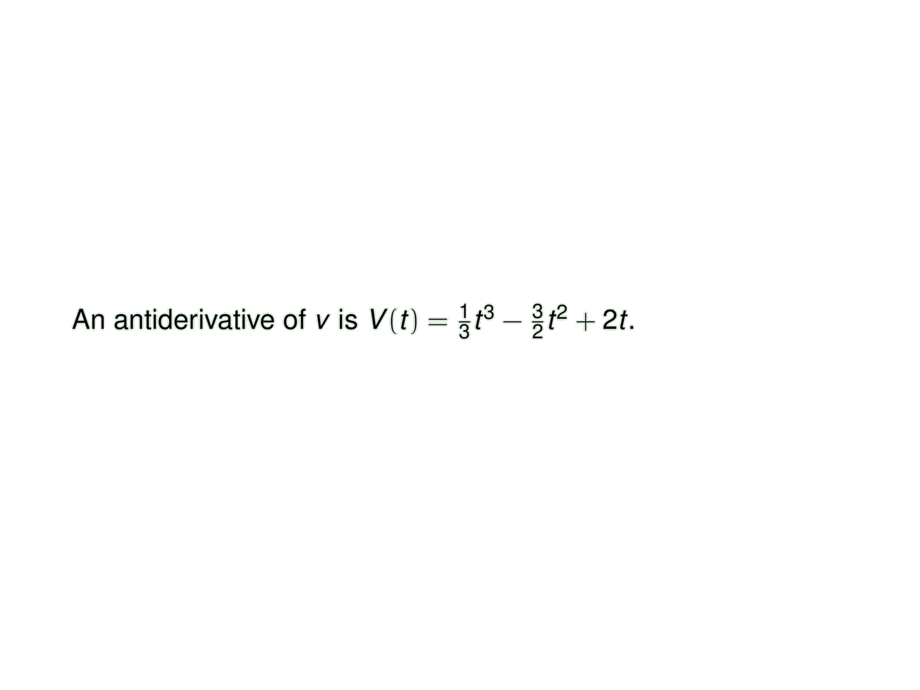





























































































54/176
\begin{frame}
\frametitle{Review - Midterm Exam 3}
\begin{exampleblock}{}
Let $v(t)$ in m/s be the velocity of a particle moving along a line.
\pause\medskip
\emph{What does $\int_0^x v(t)dt$ tell us?}
\\\pause\smallskip
Net change of the position, thus the position after $x$ seconds.
\pause\medskip
\emph{If $v(t) = t^2 - 3t + 2$, find the particles position after $1$s.}
\pause\smallskip
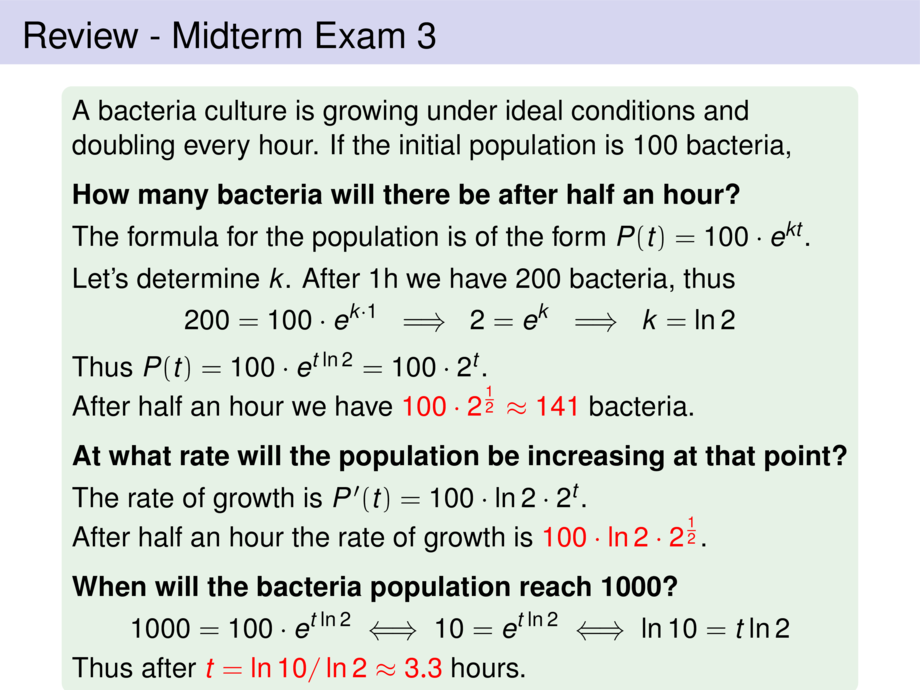
An antiderivative of $v$ is $V(t) = \frac{1}{3}t^3 - \frac{3}{2}t^2 + 2t$. \pause
Thus
\begin{talign}
p(1) = \int_0^1 v(t)dt = \mpause[1]{V(1) - V(0)} \mpause{= \frac{1}{3} - \frac{3}{2} + 2}\mpause{ = \frac{5}{6} \text{ m}}
\end{talign}
\pause\pause\pause\pause
is the position of the particle after $1$s.
\pause\medskip
\emph{What is the average velocity during the first second?}
\pause\smallskip
The average velocity is
\begin{talign}
\frac{\Delta p}{\Delta t}
\mpause[1]{= \frac{p(1)-p(0)}{1}}
\mpause{= \frac{5}{6} \text{m/s}}
\end{talign}
\end{exampleblock}
\end{frame}

