\begin{frame}
  \frametitle{The Definite Integral}

  \begin{block}{}
    The sum \quad \alert{$\sum_{i = 1}^n f(x_i) \Delta x$} \quad 
    is called \emph{Riemann sum}. 
  \end{block}
  
  \begin{center}
  \scalebox{.9}{
  \begin{tikzpicture}[default]
    \def\mfun{(-.9 + (\x-3+\mfunshift)^2 - .1*(\x-3+\mfunshift)^4)}

    \diagram[1]{-.5}{6}{-1}{1.7}{1}
    \diagramannotatez
    \def\mfunshift{0}
    \begin{scope}[ultra thick]
      \draw[cred] plot[smooth,domain=.5:5.5,samples=100] (\x,{\mfun});
%       \only<-3>{
%         \draw[draw=none,fill=cred,opacity=.5] (.5,0) -- plot[smooth,domain=.5:3.5,samples=20] (\x,{\mfun}) -- (3.5,0) -- (.5,0) -- cycle;
%       }
      \def\mwidth{5}
      \foreach \nrsteps/\mcolor in {6/cred} {
        \def\mstep{\mwidth/(\nrsteps+1)}
        \def\mfunshift{.5*\mstep}
        \foreach \xx in {0,...,\nrsteps} {
          \def\x{.5+ \xx*\mstep}
          \pgfmathparse{{\mfun}}
          \ifthenelse{\lengthtest{\pgfmathresult cm > 0cm}}{
            \def\mcolor{cgreen}
          }{}
          \draw[thick,draw=\mcolor!60!black,fill=\mcolor,opacity=.5] ({\x},0) rectangle ({\x+\mstep},{\mfun}); 
          \node[include=\mcolor] at ({\x+\mfunshift},{\mfun}) {};
        }
      }
      \node[anchor=north] at (.5,0) {$a$};
      \node[anchor=north] at (5.5,0) {$b$};
      \node[scale=1.8] at (.9,.5) {+};
      \node[scale=1.8] at (5.15,.5) {+};
      \node[scale=3] at (3,-.5) {-};
    \end{scope}
  \end{tikzpicture}
  }
  \end{center}\vspace{-1.5ex}
  
  \begin{block}{}
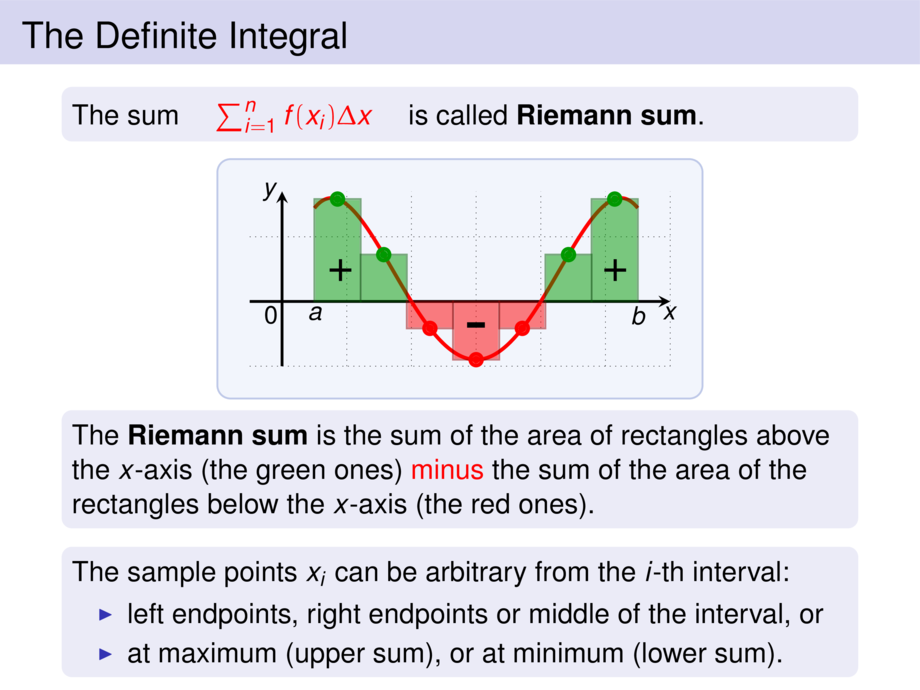
    The \emph{Riemann sum} is the sum of the area of rectangles above the $x$-axis
    (the green ones)
    \alert{minus} the sum of the area of the rectangles below the $x$-axis
    (the red ones).
  \end{block}
  \pause
  
  \begin{block}{}
  The sample points $x_i$ can be arbitrary from the $i$-th interval:
  \begin{itemize}
    \item left endpoints, right endpoints or middle of the interval, or
    \item at maximum (upper sum), or at minimum (lower sum).
  \end{itemize}
  \end{block}
 
\end{frame}