


































































































6/111
\begin{frame}
\frametitle{The Definite Integral}
\begin{block}{}
% Let $f$ be a function defined on $[a,b]$.
% \medskip
%
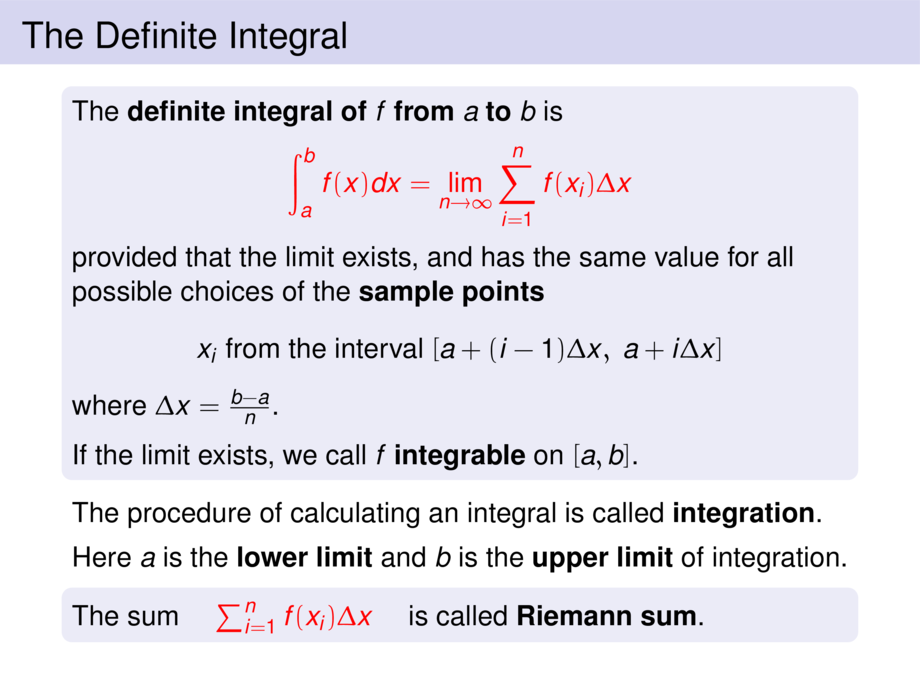
The \emph{definite integral of $f$ from $a$ to $b$} is
\begin{talign}
\alert{\int_{a}^{b} f(x)dx = \lim_{n\to \infty} \sum_{i = 1}^n f(x_i) \Delta x}
\end{talign}
provided that the limit exists, and has the same value for all possible choices
of the \emph{sample points}
\begin{center}
$x_i$ from the interval $[a + (i-1)\Delta x,\; a + i\Delta x]$
\end{center}
where $\Delta x = \frac{b-a}{n}$.\pause\medskip
If the limit exists, we call $f$ \emph{integrable} on $[a,b]$.
\end{block}
\pause\smallskip
The procedure of calculating an integral is called \emph{integration}.
\pause\medskip
Here $a$ is the \emph{lower limit} and $b$ is the \emph{upper limit} of integration.
\pause
\begin{block}{}
The sum \quad \alert{$\sum_{i = 1}^n f(x_i) \Delta x$} \quad
is called \emph{Riemann sum}.
\end{block}
\vspace{10cm}
\end{frame}

