\begin{frame}
  \frametitle{Optimization}

  \vspace{-1ex}
  \begin{exampleblock}{}
    A cylindrical can is made to hold $1$L of oil.
    Find the dimensions that minimize the cost of the metal to manufacture the can.
    \medskip\pause
    
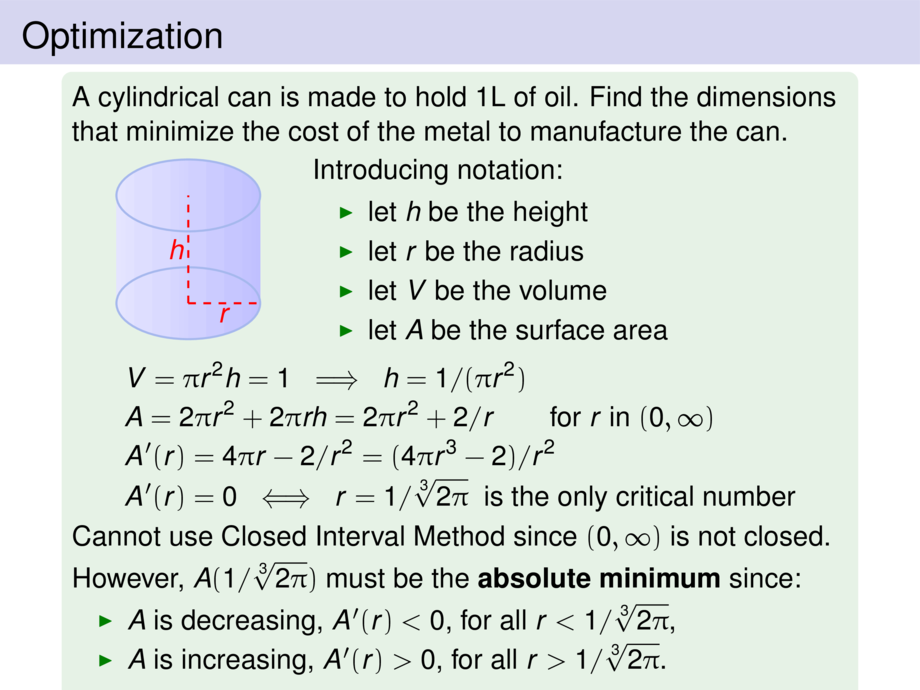
    \begin{minipage}{.3\textwidth}
    \begin{center}
      \begin{tikzpicture}[default]
        \begin{scope}[s/.style={fill=cblue!15,shade,left color=blue!10,right color=blue!25,draw=none}]
          \draw[s] (0,0) rectangle (2,1.5);
          \draw[s,draw=cblue!35] (1,0) ellipse (1 and .5);
          \draw[s,draw=cblue!35] (1,1.5) ellipse (1 and .5);
        \end{scope}
        \pause
        \begin{scope}[cred,dashed]
          \draw (1,0) -- node[left] {$h$} (1,1.5);
          \draw (1,0) -- node[below] {$r$} (2,0);
        \end{scope}
      \end{tikzpicture}
    \end{center}
    \end{minipage}
    \begin{minipage}{.69\textwidth}
      \mpause[1]{
      Introducing notation:
      \begin{itemize}
      \pause\pause
        \item let $h$ be the height
      \pause
        \item let $r$ be the radius
      \pause
        \item let $V$ be the volume
      \pause
        \item let $A$ be the surface area
      \end{itemize}
      }
    \end{minipage}\vspace{-.3ex}
    \pause    
    \mpause[0]{
    \begin{talign}
      &V = \mpause[1]{\pi r^2 h} \mpause{= 1} \mpause{\;\implies\; h = 1/(\pi r^2)} \\[-.5ex]
      &\mpause{A = }\mpause{2\pi r^2 + 2\pi r h} \mpause{= 2\pi r^2 + 2/r} \quad\quad \text{\mpause{for $r$ in }\mpause{$(0,\infty)$}} \\[-.5ex]
      &\mpause{A'(r) = }\mpause{4\pi r - 2/r^2} \mpause{= (4\pi r^3 - 2)/r^2} \\[-.5ex]
      &\mpause{A'(r) = 0 \;\iff\; r = }\mpause{1/\sqrt[3]{2\pi}}
      \mpause{\text{\ \ is the only critical number}}
    \end{talign}\vspace{-3ex}
    \pause\pause\pause\pause\pause\pause\pause\pause\pause\pause\pause\pause\pause\pause\pause
    
    Cannot use Closed Interval Method since $(0,\infty)$ is not closed.
    \pause\smallskip
    
    However, $A(1/\sqrt[3]{2\pi})$ must be the \emph{absolute minimum}\only<-27>{ since:
    \begin{itemize}
    \pause
      \item $A$ is decreasing, $A'(r) < 0$, for all $r < 1/\sqrt[3]{2\pi}$,  
     \pause
      \item $A$ is increasing, $A'(r) > 0$, for all $r > 1/\sqrt[3]{2\pi}$.
    \end{itemize}}
    \pause\pause\pause\pause\smallskip
    
    Then $h = 1/(\pi r^2) \pause= \sqrt[3]{2\pi}^2/\pi \pause= \sqrt[3]{4\pi^2/\pi^3} \pause= 2 / \sqrt[3]{2\pi} \pause= 2r$
    \pause\smallskip
    
    Hence \alert{radius $r = 1/\sqrt[3]{2\pi}$} and \alert{height $h = 2r$} minimizes the cost. 
    }
  \end{exampleblock}
  \vspace{10cm}
\end{frame}