


































































































12/118
\begin{frame}
\frametitle{L'Hospital's Rule}
\begin{block}{L'Hospital's Rule}
Suppose $f$ and $g$ are differentiable and $g'(x) \ne 0$ near $a$, and
\begin{talign}
\lim_{x\to a} \frac{f(x)}{g(x)}
\end{talign}
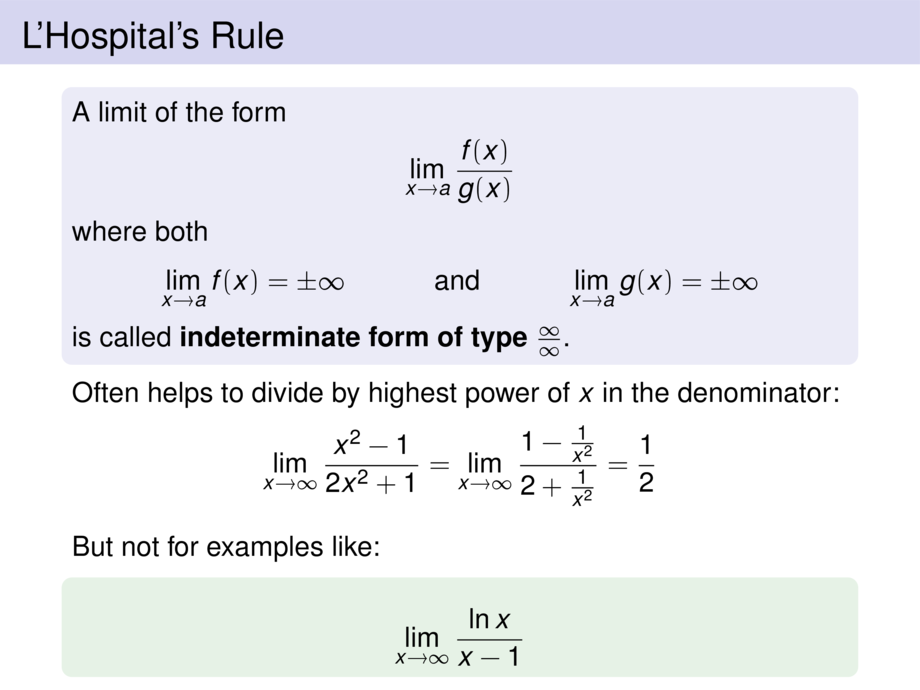
is an indeterminate form of type \alert<3->{$\frac{0}{0}$} or \alert<3->{$\frac{\infty}{\infty}$}.
Then
\begin{talign}
\lim_{x\to a} \frac{f(x)}{g(x)} = \lim_{x\to a} \frac{f'(x)}{g'(x)}
\end{talign}
if the limit on the right side exists or is $-\infty$ or $\infty$.
\end{block}
\pause
(near $a$ = on an open interval containing $a$ except possibly $a$ itself)\hspace*{-20ex}
\pause\bigskip
\alert{Before applying L'Hospital's Rule it is important to verify that:}\vspace{-.25ex}
\begin{exampleblock}{}\vspace{-1.1ex}
\begin{malign}
\lim_{x \to a} f(x) = 0 \text{\quad and \quad} \lim_{x \to a} g(x) = 0
\end{malign}\vspace{-.5ex}
\end{exampleblock}\vspace{-.8ex}
or\vspace{-.7ex}
\begin{exampleblock}{}\vspace{-1.1ex}
\begin{malign}
\lim_{x \to a} f(x) = \pm\infty \text{\quad and \quad} \lim_{x \to a} g(x) = \pm\infty
\end{malign}\vspace{-.5ex}
\end{exampleblock}
\end{frame}

