\begin{frame}
  \frametitle{Continuity}

  \begin{block}{}
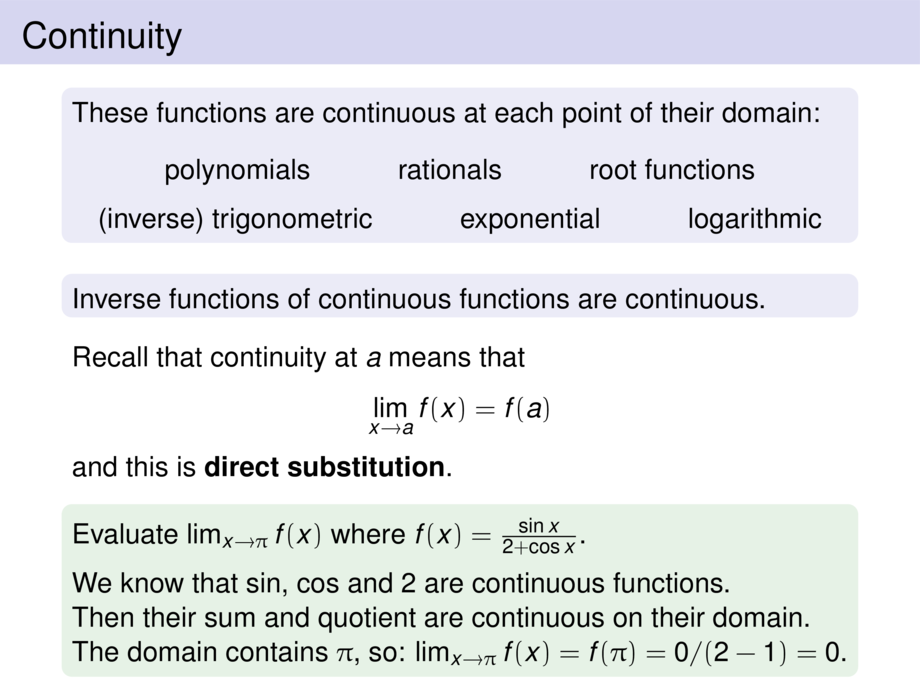
    These functions are continuous at each point of their domain:
    \begin{center}
      polynomials \hspace{1cm} rationals \hspace{1cm} root functions\\[1ex]     
      (inverse) trigonometric \hspace{1cm} exponential \hspace{1cm} logarithmic      
    \end{center}
  \end{block}
  \pause\medskip
  
  \begin{block}{}
    Inverse functions of continuous functions are continuous.
  \end{block}  
  \pause\medskip

  Recall that continuity at $a$ means that 
  \begin{align*}
    \lim_{x\to a} f(x) = f(a)
  \end{align*}
  and this is \emph{direct substitution}.
  \pause\medskip
  
  \begin{exampleblock}{}
    Evaluate $\lim_{x\to \pi} f(x)$ where $f(x) = \frac{\sin x}{2 + \cos x}$. \pause\\[1ex]
    We know that $\sin$, $\cos$ and $2$ are continuous functions.\\\pause
    Then their sum and quotient are continuous on their domain.\\\pause
    The domain contains $\pi$, so: $\lim_{x\to \pi} f(x) = f(\pi) = 0/(2-1) = 0$.
  \end{exampleblock}
\end{frame}