\begin{frame}
  \frametitle{Continuity: Examples}
  
  \begin{block}{}
    A function $f$ is \emph{continuous form the right} at a number $a$ if
    \begin{talign}
      \lim_{x\to a^+} f(x) = f(a)
    \end{talign}
  \end{block}
  \pause
  \begin{block}{}
    A function $f$ is \emph{continuous form the left} at a number $a$ if
    \begin{talign}
      \lim_{x\to a^-} f(x) = f(a)
    \end{talign}
  \end{block}
  \pause\smallskip
  
  \begin{minipage}{.58\textwidth}
  \begin{exampleblock}{}
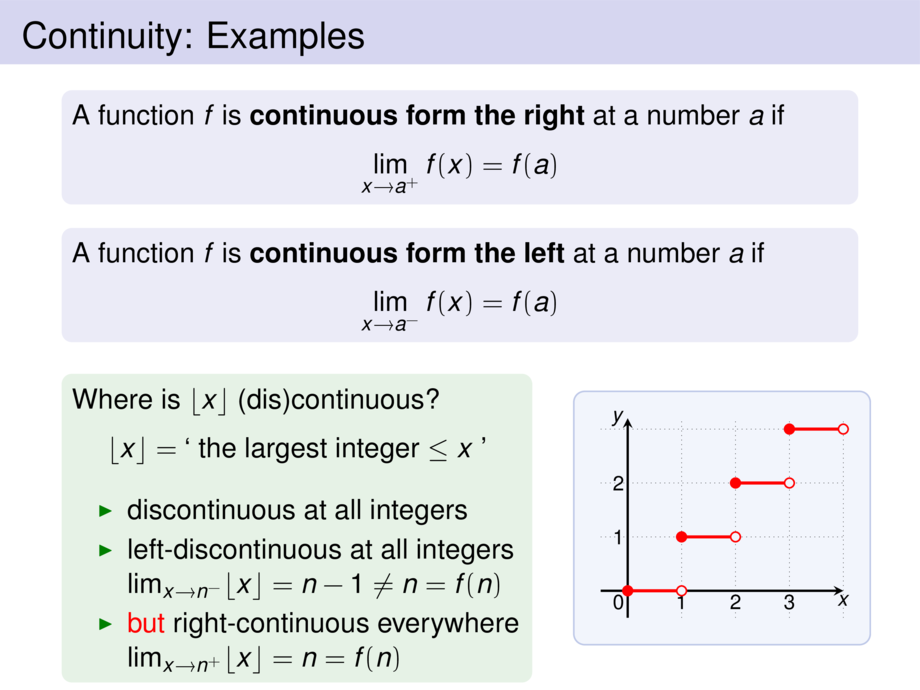
    Where is $\lfloor x \rfloor$ (dis)continuous?
    \begin{talign}
      \lfloor x \rfloor = \text{`\;the largest integer $\le x$\;'}
    \end{talign}\vspace{-2ex}
    \begin{itemize}
      \item<4-> discontinuous at all integers
      \item<5-> left-discontinuous at all integers\\
        $\lim_{x\to n^-} \lfloor x \rfloor = n-1 \ne n = f(n)$
      \item<6-> \alert{but} right-continuous everywhere\\
        $\lim_{x\to n^+} \lfloor x \rfloor = n = f(n)$
    \end{itemize}
  \end{exampleblock}
  \end{minipage}~\quad~
  \begin{minipage}{.39\textwidth}
    \scalebox{.75}{
    \begin{tikzpicture}[default]
      \diagram{-.5}{4}{-.5}{3.2}{1}
      \diagramannotatez
      \diagramannotatex{1,2,3}
      \diagramannotatey{1,2}
      \foreach \x in {0,1,2,3} {
        \draw[cred,ultra thick] (\x,\x) -- ++(1,0);
        \node[include={cred}] at (\x,\x) {};
        \node[exclude={cred}] at (\x+1,\x) {};
      }
    \end{tikzpicture}
    }
  \end{minipage}
\end{frame}