\begin{frame}
  \frametitle{Exponential Functions: Irrational Numbers}

  \begin{alertblock}{}
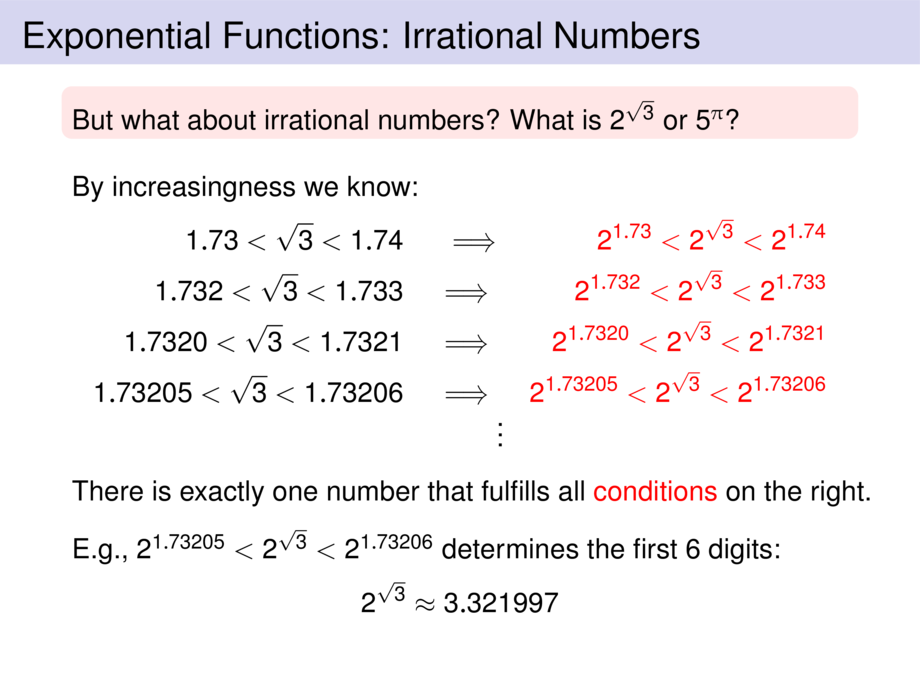
    But what about irrational numbers?
    What is $2^{\sqrt{3}}$ or $5^\pi$?
  \end{alertblock}
  \bigskip
  \pause
  
  By increasingness we know:
  \begin{talign}
    1.73 < \sqrt{3} < 1.74 &&\implies&& \alert<7->{2^{1.73} < 2^{\sqrt{3}} < 2^{1.74}} \\
    \mpause[1]{1.732 < \sqrt{3} < 1.733} &&\mpause[1]{\implies}&& \mpause[1]{\alert<7->{2^{1.732} < 2^{\sqrt{3}} < 2^{1.733}}} \\
    \mpause[2]{1.7320 < \sqrt{3} < 1.7321} &&\mpause[2]{\implies}&& \mpause[2]{\alert<7->{2^{1.7320} < 2^{\sqrt{3}} < 2^{1.7321}}} \\
    \mpause[3]{1.73205 < \sqrt{3} < 1.73206} &&\mpause[3]{\implies}&& \mpause[3]{\alert<7->{2^{1.73205} < 2^{\sqrt{3}} < 2^{1.73206}}} \\[-1ex]
    && \mpause[4]{\vdots} &&
  \end{talign}
  \pause[7]\vspace{-2ex}
  
  There is exactly one number that fulfills all \alert{conditions} on the right.\hspace*{-.5cm}
  \pause\bigskip
  
  E.g., $2^{1.73205} < 2^{\sqrt{3}} < 2^{1.73206}$ determines the first $6$ digits:
  \begin{talign}
    2^{\sqrt{3}} \approx 3.321997
  \end{talign}
  \vspace{10cm}
\end{frame}